Mobile Notice
You appear to be on a device with a "narrow" screen width (i.e. you are probably on a mobile phone). Due to the nature of the mathematics on this site it is best viewed in landscape mode. If your device is not in landscape mode many of the equations will run off the side of your device (you should be able to scroll/swipe to see them) and some of the menu items will be cut off due to the narrow screen width.
Section 12.5 : Functions of Several Variables
6. Identify and sketch the level curves (or contours) for the following function.
\[4z + 2{y^2} - x = 0\]Show All Steps Hide All Steps
Start SolutionWe know that level curves or contours are given by setting \(z = k\). Doing this in our equation gives,
\[4k + 2{y^2} - x = 0\] Show Step 2A quick rewrite of the equation from the previous step gives us,
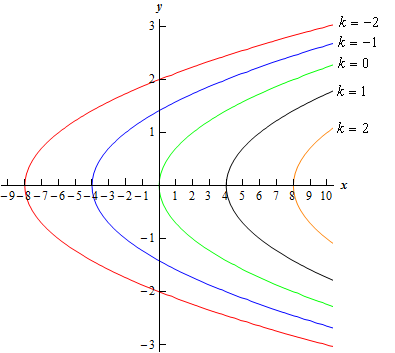
\[x = 2{y^2} + 4k\]So, the level curves for this function will be parabolas opening to the right and starting at 4\(k\).
Note as well that there will be no restrictions on the values of \(k\) that we can use, as there sometimes are.
Show Step 3Below is a sketch of some level curves for some values of \(k\) for this function.